Why is mathematics considered difficult ?

By R.N.A. de Silva

If a survey is carried out amongst students in a school to find out the subject which causes most difficulties, there is no doubt the majority would say that it is mathematics. Most parents too are of the opinion that this is a subject regarding which they are unable to help their children. Why has this situation arisen? What can be done to change this?

It needs to be understood that mathematics is different from other subjects in the school curriculum. It requires a new language to be learnt and a myriad of symbols to be manipulated. In addition, the thought processes are different, and they are subject to rigid rules. Another important factor is that the objective of learning a concept is not readily observed until a certain standard is achieved. For example, in algebra, algebraic manipulation needs to be mastered before applying it to real life situations such as optimization. Unlike in most other subjects, visual verification or experimentation is problematic in mathematics due to the abstractness of the concepts. Learning mathematics involves connected thinking along various mathematical topics and ideas, and accuracy of thought and the writing process. Therefore, a good memory also plays an important role. To be a good mathematician, the ability to recognize patterns and the power of generalization need to be cultivated and enhanced.

Consider the following example, where the number of triangles formed by matchsticks are gradually increased.

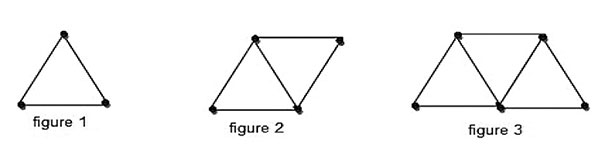
Through observation, the following can be obtained.

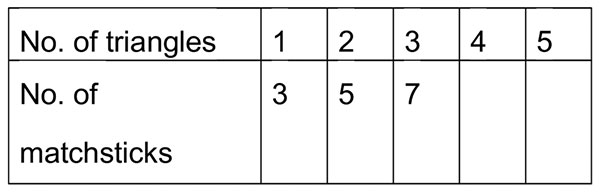
At the upper primary level, students should have the ability to figure out that the number of matchsticks required to form four and five (or higher) number of triangles are nine and 11, by following the pattern. At secondary level they should be able to generalize it with a formula such as “if the number of triangles is ‘n’, then the number of matchsticks should be ‘2n+1’”. Mathematics involves the search for patterns.

The difficulties that students experience in learning mathematics and thereby flunking examinations can be due to following reasons.

1. Negative attitude

Our attitude is what influences all our actions. It is only the right attitude, which gets us good results. Unfortunately, some students give up easily without taking up the challenges math offers thinking that they are unable to deal with abstract concepts. Gradually they will develop a phobia for the subject. This in turn will affect learning ability and performance. Another factor that may influence a negative attitude is peer pressure. Constant comparison with students who are performing well may lead to negative feelings about self-ability. It is difficult to recover if self-doubt sets in.

Teachers and parents have an important role to play in students cultivating a positive attitude towards mathematics. There is a need to understand that different people may work at different pace, in differing situations. The pace of working may gradually enhance with practice and good conceptual understanding. Therefore, comparisons may not always bring positive results. Appropriate commendation and encouragement by teachers and parents will go a long way in building a positive attitude.

2. Aloofness of subject

Many students find it difficult to develop a connection between the subject and themselves as they are unable to comprehend the reasons for studying a given topic. They treat it as something mysterious and frightening. This may hamper learning ability. Such students may get easily distracted. For example, in secondary school a substantial amount of time is spent on the procedures of solving quadratic equations. The knowledge of quadratics is required in situations such as when gauging the motion of a particle under gravity, finding distances covered by a vehicle, maximizing profit or minimizing cost, but it is not possible to deal with these applications until quadratic equations are mastered.

As far as possible, teachers should try to connect mathematical concepts to real life problems and establish connections between various forms of mathematical knowledge. The Principles and Standards for School Mathematics produced by the National Council of Teachers of Mathematics has recommended that the mathematics instruction should enable students to:

Recognize and use connection among mathematical ideas

Understand how mathematical ideas interconnect and build on one another to produce a coherent whole

Recognize and apply mathematics in contexts outside of the mathematical field.

Connecting mathematics to real life experiences as well as other subject areas will help students to understand the relevance of their learning material.

3. Confusion with signs and symbols

Mathematics is a symbolic language. The key to comprehending mathematics is in the interpretation of the concepts. But to be able to understand the concepts a good awareness of the signs and symbols is essential. There should be an appreciation of their usefulness in making mathematical statements simpler and elegant. A good grasp of the exact meaning of these signs and symbols and the role they play is a prerequisite for success. A common mistake is to take them for granted and not pay too much attention to them. However, it has to be kept in mind that sometimes it is the little things that are the most important. These signs and symbols provide a universally perceivable system to express mathematical statements and their significance should be properly understood. Some of the common symbols are given below.

Care must be taken when the same symbol can be used in different situations. For example, 3.5 is a decimal number read as ‘three point five’. The dot as the decimal marker is written on the baseline and it is not surrounded by spaces. However, 3 5 represents the product of 3 and 5 read as ‘three times five’. The dot for multiplication is usually written raised up at the central level and surrounded by spaces. Similarly, the ‘minus’ sign in the statement 7 – 5 represents the subtraction of 5 from 7, which is a mathematical operation. However, when it is placed before a numeral such as -3, it represents the negative number three, which is a property of a number. Sometimes, a raised minus sign is used to denote negative numbers.

4. Inadequate practice

As mathematics is a subject that builds upon previously learnt concepts, practice is important to gain a deeper understanding of the procedures of problem solving, making connections between concepts, and engaging in thinking mathematically. Practice needs to involve not only skill enhancement but also thinking and reasoning to sustain motivational learning.

Kilpatrick, Swafford and Findell in their research report ‘Adding it up: Helping children learn mathematics’ identified five strands of mathematical proficiency:

Procedural fluency: Skill in carrying out procedures flexibly, accurately, efficiently and appropriately

Strategic competence: Ability to formulate, represent and solve mathematical problems

Adaptive reasoning: Capacity for logical thought, reflection, explanation and justification

Conceptual understanding: Comprehension of mathematical concepts, operations and relations

Productive disposition: Habitual inclination to see mathematics as sensible, useful and worthwhile, coupled with a belief in diligence and one’s own efficacy.

It is important to reflect on the solutions at the conclusion of the problem solving process, which many of our students lack. For example, consider the following problems.

Four men build a wall in 12 days. How long would it take six similar men? Consider coming up with a solution of 18 days. Is this sensible? If you have more workers, the job should be completed sooner. Therefore 18 cannot be the answer.

The average number of matchsticks is 99.5 in 4 boxes of matches. When a fifth box is added, the average of the five boxes becomes 100. How many matches are there in the fifth box? If a student comes up with a solution of 102.5, on reflection he or she should realize that this is not correct as the number of matchsticks has to be a whole number.

Teachers have an important role to play in all the above factors, in creating a comfortable environment to learn the subject with interest and considering the students’ academic capabilities and social and psychological status in dealing with the difficulties that students face so that appropriate action can be taken to keep the motivational levels high. In George Polya’s words “To teach effectively a teacher must develop a feeling for his subject; he cannot make his students sense its vitality if he does not sense it himself. He cannot share his enthusiasm when he has no enthusiasm to share. How he makes his point may be as important as the point he makes; he must personally feel it to be important.”

As parents are children’s first role models, it is important for parents to ensure that a mutually supportive framework is provided at home for children’s mathematical education. This can be done by showing an interest in what their children are learning and fostering positive attitudes towards mathematics. Encouraging children to cultivate perseverance when solving problems and acknowledging difficulties will help build their self-confidence and motivation. Let them enjoy the joy of losing themselves and their cares in a problem that taxes their minds.

(The author is a senior examiner for mathematics in the International Baccalaureate Organization and a member of the faculty of the Overseas School of Colombo.)